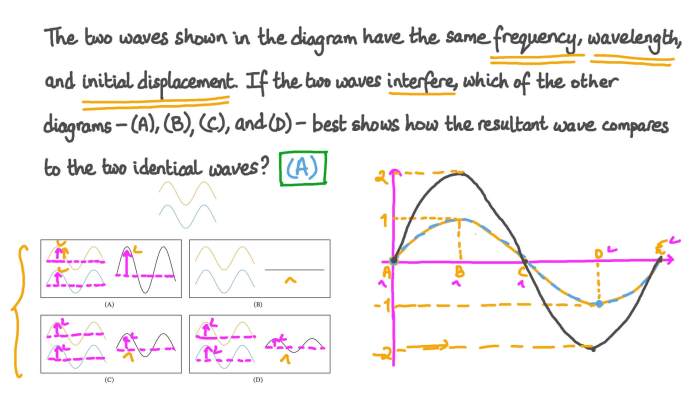
When two waves combine, the resulting wave’s amplitude is influenced by the amplitudes of the original waves. If the amplitude of the resultant wave is twice that of the original waves, this phenomenon offers valuable insights into wave interactions and has practical applications across various disciplines.
Delving into the concept of wave amplitude and the formation of resultant waves, this exploration examines the factors that determine the amplitude of the resultant wave. By understanding these principles, we gain a deeper comprehension of wave behavior and its significance in fields ranging from acoustics to engineering.
Wave Amplitude
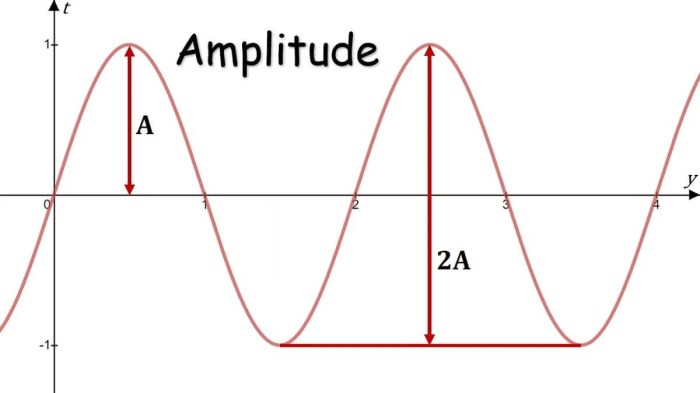
Wave amplitude refers to the maximum displacement of a wave from its equilibrium position. It determines the height of the wave crest and the depth of the wave trough. Amplitude is typically measured in units of distance, such as meters or centimeters.
Waves with different amplitudes can be distinguished visually. For instance, a wave with a larger amplitude will have a higher crest and a deeper trough compared to a wave with a smaller amplitude.
Resultant Wave
A resultant wave is a wave that results from the combination of two or more individual waves. When waves interact, they can either reinforce or cancel each other out, depending on their relative phases.
If the waves are in phase, they reinforce each other, resulting in a resultant wave with an amplitude that is greater than the amplitudes of the individual waves. If the waves are out of phase, they cancel each other out, resulting in a resultant wave with an amplitude that is smaller than the amplitudes of the individual waves.
Amplitude of Resultant Wave
The amplitude of the resultant wave depends on the amplitudes and phases of the individual waves that combine to form it. If the individual waves have the same amplitude and are in phase, the amplitude of the resultant wave will be twice the amplitude of the individual waves.
For example, if two waves with an amplitude of 1 meter are in phase, the resultant wave will have an amplitude of 2 meters.
Factors Affecting Amplitude, If the amplitude of the resultant wave is twice
Several factors can affect the amplitude of a wave, including the energy of the wave, the medium through which the wave is traveling, and the presence of obstacles or boundaries.
Waves with higher energy typically have larger amplitudes. The medium through which a wave travels can also affect its amplitude. For instance, waves traveling through a denser medium will have a smaller amplitude compared to waves traveling through a less dense medium.
Obstacles or boundaries can also affect the amplitude of a wave. When a wave encounters an obstacle, it can be reflected, refracted, or absorbed. These interactions can lead to changes in the wave’s amplitude.
Applications
Understanding the amplitude of the resultant wave is important in various applications, including acoustics, optics, and engineering.
In acoustics, the amplitude of the resultant wave determines the loudness of a sound. In optics, the amplitude of the resultant wave determines the brightness of light. In engineering, the amplitude of the resultant wave is considered when designing structures that are subjected to vibrations or waves.
General Inquiries: If The Amplitude Of The Resultant Wave Is Twice
What is the significance of the amplitude of a resultant wave?
The amplitude of the resultant wave provides insights into the strength and intensity of the wave, as it represents the maximum displacement of the wave from its equilibrium position.
How does the amplitude of the resultant wave relate to the amplitudes of the original waves?
The amplitude of the resultant wave is influenced by the amplitudes of the original waves. In certain scenarios, such as constructive interference, the resultant wave’s amplitude can be twice that of the original waves.
What are some practical applications of understanding the amplitude of the resultant wave?
Understanding the amplitude of the resultant wave has applications in various fields, including acoustics, optics, and engineering. It enables us to design and optimize systems that utilize wave phenomena, such as soundproofing materials, optical devices, and wave-based technologies.